Pick's Rule

  To find out what Pick's rule is do the discovery activity below.

Lattice Grid Points and Area
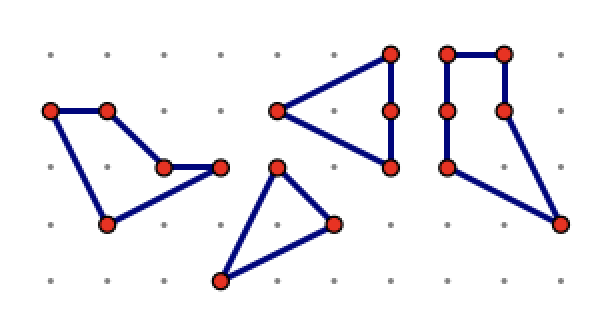
Part 1: Below are some polygons with no points in the Interior (I) and the number of border points (B) varies. Find the areas of each polygon and enter your results in the table.




B
 I
 Area
3
 0
 1/2
4
 0
 1
5
 0
 1 1/2
6
 0
 2
7
 0
 2 1/2










Question: What would be the area of a polygon that had zero inside points and 15 boundary points?

The student may extend the table to get the answer 6 1/2.

What did you do to figure out the area for 15 boundary points? Could you have used a rule (in equation form) to find the answer?

The equation for the areas above equals B/2 - 1. So we substitute 15 for B and get 15/2 - 1 = 6 1/2 square units.
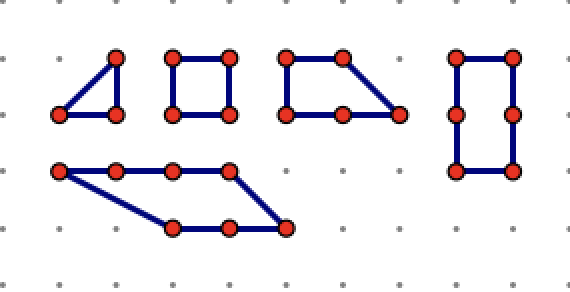
Part 2: Below are some polygons that have 1 interior point. What is the rule (equation) connecting B and Area for these shapes?

Area = B/2
B
 I
 Area
3
 1
 1 1/2
4
 1
 2
5
 1
 2 1/2
6
 1
 3
7
 1
 3 1/2









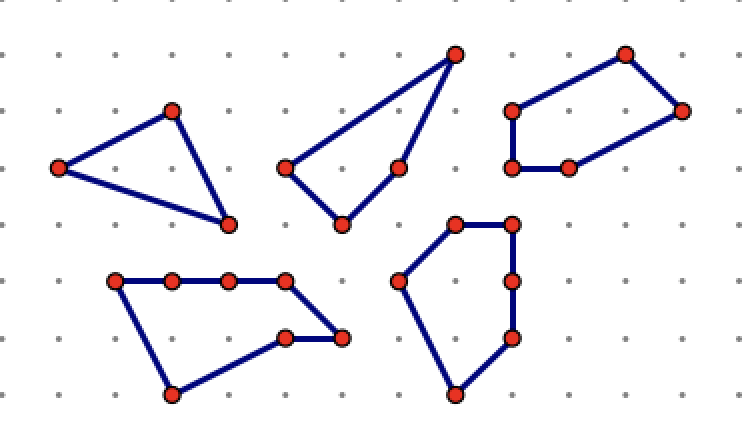
Part 3: Below are some polygons that have 2 interior points. What is the rule ( equation) connecting B and Area for these shapes?

Area = B/2 + 1
B
 I
 Area
3
 2
 2 1/2
4
 2
 3
5
 2
 3 1/2
6
 2
 4
7
 2
 4 1/2









Part 4: Summarize your findings from the three tables above. What conclusions do you draw? Can you write an equation that lets you find the area (A) from the number of points on the boundary (B) and points inside (I)?

In summary:
B
 I
 Area
3
 0
 1/2
4
 0
 1
5
 0
 1 1/2
6
 0
 2
7
 0
 2 1/2
B
 I
 Area
3
 1
 1 1/2
4
 1
 2
5
 1
 2 1/2
6
 1
 3
7
 1
 3 1/2
B
 I
 Area
3
 2
 2 1/2
4
 2
 3
5
 2
 3 1/2
6
 2
 4
7
 2
 4 1/2

When I = 0 Area = B/2 - 1
When I = 1 Area = B/2 + 0
When I = 2 Area = B/2 + 1

Note the relationship between I and the number added to B/2:

When I = 0 number added to B/2 = -1
When I = 1 number added to B/2 = 0
When I = 2 number added to B/2 = 1

Note the number added to B/2 is 1 less than I. So the rule can be written like this:

Area = B/2 + I - 1

which is Pick's Law.